1966 Activity: Inverse Square Law

The inverse square law states that a light source which is twice as far away appears one fourth as bright.  A light that is three times as far away is one ninth as bright.  In other words, apparent brightness diminishes with the square of the distance. 

 

If you happen to have a light meter you can test the inverse square law by turning on a bare bulb in a dark room.  Hold a white card one meter away from the bulb and direct your light meter toward the card. Now do the same with the card two meters away, three meters, and so on.  How close are the resulting readings to the predicted readings?

 

Few people have light meters these days since most cameras have built-in meters that work automatically.  However you can convince yourself of the inverse square law by imagining how light would spread out.  Look at the sketch below.

 

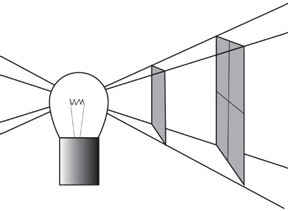Assume the light spreads out equally in all directions.  At a distance of one foot from the filament the light strikes a card one foot in diameter.  At a distance of two feet, how many cards are necessary to catch the same rays of light?  How does the intensity of light on each card compare with the intensity of light on the card just one foot away?